NCERT/CBSE
- CBSE Class 10 Syllabus
- NCERT Class 10 Solutions

https://www.urbanpro.com/ncert-solutions/class-10/maths-pair-linear-equations-two-variable-exercise-3-2

Learn Exercise 3.2 with Free Lessons & Tips

Question 1:

Which of the following pairs of linear equations are consistent/inconsistent? If consistent, obtain the solution graphically:

(i) x + y = 5, 2x + 2y = 10 (ii) x – y = 8, 3x – 3y = 16 (iii) 2x + y – 6 = 0, 4x – 2y – 4 = 0 (iv) 2x – 2y – 2 = 0, 4x – 4y – 5 = 0

Solution 1:

$(i)$ Here, $\frac{a_{1}}{a_{2}}= \frac{1}{2}$ , $\frac{b_{1}}{b_{2}}= \frac{1}{2}, \frac{c_{1}}{c_{2}}= \frac{-5}{-10}$

$\therefore \frac{a_{1}}{a_{2}}=\frac{b_{1}}{b_{2}}=\frac{c_{1}}{c_{2}}$

Thus, the pair of linear equations is consistent.
Now, x + y = 5 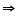x = 5 - y

x	4	3	2
y	1	2	3

$2x+2y=10$

$x=\frac{10-2y}{2}$

x	4	3	2
y	1	2	3

From the graph, it can be observed that the two lines coincide. Thus, the given pair of equations has infinite solutions.
Let x = k, then y = 5 - k. So, the ordered pair (k, 5 - k) , where k is a constant, will be the solution of the given pair of linear equations.

$(ii)$ Here, $\frac{a_{1}}{a_{2}}= \frac{1}{3}, \frac{b_{1}}{b_{2}}=\frac{1}{3}, \frac{c_{1}}{c_{2}}= \frac{1}{2}$

$\therefore \frac{a_{1}}{a_{2}}=\frac{b_{1}}{b_{2}} eq \frac{c_{1}}{c_{2}}$

Thus, the pair of linear equations is inconsistent. Hence the lines appear parallel.

$(iii)$ $\frac{a_{1}}{a_{2}}= \frac{1}{2}, \frac{b_{1}}{b_{2}}=\frac{-1}{2}, \frac{c_{1}}{c_{2}}=\frac{3}{2}$

since $\frac{a_{1}}{a_{2}} eq \frac{b_{1}}{b_{2}}$ , the equation has only one solution.

Thus, the pair of linear equations is consistent.

Now, 2x + y - 6 = 0 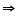y = 6 - 2x

x	0	1	2
y	6	4	2

$4x-2y-4=o$

$y=\frac{4x-4}{2}$

x	1	2	3
y	0	2	4

From the graph, it can be observed that the two lines intersect each other at the point (2, 2). Thus, the solution of the given pair of equations is (2, 2).

$(iv)$ $\frac{a_{1}}{a_{2}}=\frac{1}{2}, \frac{b_{1}}{b_{2}}=\frac{1}{2}, \frac{c_{1}}{c_{2}}= \frac{2}{5}$

$\therefore \frac{a_{1}}{a_{2}}=\frac{b_{1}}{b_{2}} eq \frac{c_{1}}{c_{2}}$

The equation has no solution.

Thus, the pair of linear equations is inconsistent.

Comments

Tasneem

Question 2:

Half the perimeter of a rectangular garden, whose length is 4 m more than its width, is 36 m. Find the dimensions of the garden.

Solution 2:

Let the width of the garden = x m

Length of the garden = x+4 m

Given, half of the perimeter =36 m

=> l+b = 36

=> x+x+4=36

2x+4=36

2x=36-4

2x=32

x =32/2 = 16

Therefore, width of the garden =16 m

Length of the garden = 16+4=20 m

According to the given conditions, for graphical representation,
y - x = 4
y + x = 36

y - x = 4 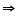y = x + 4

x	0	8	12
y	4	12	16

y + x = 36

x	0	36	16
y	36	0	20

From the graph, it can be observed that the two lines intersect each other at the point (16, 20). So, x = 16 and y = 20.
Thus, the length and width of the rectangular garden is 20 m and 16 m respectively.

Comments

Bhavani Ramesh

Question 3:

Given the linear equation 2x + 3y – 8 = 0, write another linear equation in two variables such that the geometrical representation of the pair so formed is: (i) intersecting lines (ii) parallel lines (iii) coincident lines

Solution 3:

(i)For intersecting lines,

$\frac{2}{a} eq \frac{3}{b} eq \frac{8}{c}$ where a, b, c can have any value which satisfy the above equation

Let a=3, b=2 and c= 4

$\therefore 2v+3y=8$ and $3a+2y=4$ give unique solutions and their geographic representation shows intersecting points.

(ii) For parallel lines,

$\frac{2}{a} eq \frac{3}{b} eq \frac{8}{c}$

where a, b, c can have any value which satisfy the above equation.

Let a=2, b=3 and c= 4

the equation has no solution and the geometrical representation shows parallel lines.

(iii) For coincident lines,

$\frac{2}{3}=\frac{3}{2}=\frac{8}{4}$ where a, b, c can have any value which satisfy the above equation

Let a=4, b=6 and c= 16

the required equation will be $4x+6y=16$

the equation has infinitely many solutions and their geographical representation shows coincident lines.

Comments

Tasneem

Question 4:

Draw the graphs of the equations x – y + 1 = 0 and 3x + 2y – 12 = 0. Determine the coordinates of the vertices of the triangle formed by these lines and the x-axis, and shade the triangular region.

Solution 4:

Line 1

x-y+1=0

Coordinates

x=0 y=1 (0,1)

y=0 x= -1 (-1,0)

x=2, y=3 (2,3)

Line 2

3x+2y-12=0

x=0, y=6 (0,6)

y=0 x=4 (4,0)

x=2,y=3 (2 ,3)

Both lines can be drawn with the details of cordinates.

The vertices of the triangles

are B(-1,0),C (4,0) and A (2,3)

Comments

Venkatesan Iyer

Question 5:

Form the pair of linear equations in the following problems, and find their solutions graphically.

(i) 10 students of Class X took part in a Mathematics quiz. If the number of girls is 4 more than the number of boys, find the number of boys and girls who took part in the quiz.

(ii) 5 pencils and 7 pens together cost Rs. 50, whereas 7 pencils and 5 pens together cost Rs. 46. Find the cost of one pencil and that of one pen.

Solution 5:

(i) Let the number of girls and boys in the class be x and y respectively.
According to the given conditions, we have:
x + y = 10
x - y = 4
x + y = 10 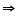x = 10 - y

x	5	4	6
y	5	6	4

$x-y=4$

$x=4+y$

x	5	4	3
y	1	0	-1

The graphical representation is as follows:

From the graph, it can be observed that the two lines intersect each other at the point (7, 3).
So, x = 7 and y = 3.

Thus, the number of girls and boys in the class are 7 and 3 respectively.

(ii) Let the cost of one pencil and one pen be Rs x and Rs y respectively.

According to the given conditions, we have:
5x + 7y = 50
7x + 5y = 46

$5x+7y=50$

$x=\frac{50-7y}{5}$

x	3	10	-4
y	5	0	10

$7x+5y=46$

$x=\frac{46-5y}{7}$

x	8	3	-2
y	-2	5	12

From the graph, it can be observed that the two lines intersect each other at the point (3, 5).
So, x = 3 and y = 5.

Therefore, the cost of one pencil and one pen are Rs 3 and Rs 5 respectively.

Comments

Tasneem

Question 6:

On comparing the ratios $\frac{a_{1}}{^{a_{2}}}$ , $\frac{b_{1}}{b_{2}}$ , and $\frac{c_{1}}{c_{2}}$ , find out whether the lines representing the following pairs of linear equations intersect at a point, are parallel or coincident:

(i) $5x-4y+8=0, 7x+6y-9=0$

(ii) $9x+3y+12=0, 18x+6y+24=0$

(iii) $6x-3y+10=0, 2x-y+9=0$

Solution 6:

(i) 5x - 4y + 8 = 0
7x + 6y - 9 = 0
Comparing these equations with a₁x + b₁y + c₁ = 0 and a₂x + b₂y + c₂ = 0, we get:

$a_{1}= 5, b_{1}=-4, c_{1} =8$

$a_{2}= 7, b_{2}= 6, c_{2}= -9$

$\frac{a_{1}}{a_{2}}= \frac{5}{7}, \frac{b_{1}}{b_{2}}= \frac{-2}{3}$

Since, $\frac{a_{1}}a_{2}{} eq \frac{b_{1}}{b_{2}}$ the given pair of equations intersect at exactly one point.

(ii) 9x + 3y + 12 = 0
18x + 6y + 24 = 0
Comparing these equations with a₁x + b₁y + c₁ = 0 and a₂x + b₂y + c₂ = 0, we get:

$a_{1}=9, b_{1}=3, c_{1}=12$

$a_{2}=18, b_{2}=6, c_{2}=24$

$\frac{a_{1}}{a_{2}}=\frac{9}{18}=\frac{1}{2}$

$\frac{b_{1}}{b_{2}}= \frac{3}{6}=\frac{1}{2}$

$\frac{c_{1}}{c_{2}}= \frac{12}{24}=\frac{1}{2}$

Since $\frac{a_{1}}a_{2}{=\frac{b_{1}}b_{2}{}}=\frac{c_{1}}{c_{2}}$

the given pair of equation are coincident.

(iii) 6x - 3y + 10 = 0 , 2x - y + 9 = 0

Comparing these equations with a₁x + b₁y + c₁ = 0 and a₂x + b₂y + c₂ = 0, we get:

$a_{1}=6, b_{1}=-3, c_{1}=10$

$a_{2}=2, b_{2}=-1,c_{2}=9$

$\frac{a_{1}}a_{2}{=\frac{6}{2}}=\frac{3}{2}$

$\frac{b_{1}}{b_{2}}= \frac{-3}{-1}=\frac{3}{1}$

$\frac{c_{1}}c_{2}{}=\frac{10}{9}$

Since, $\frac{a_{1}}{a_{2}}=\frac{b_{1}}{b_{2}} eq \frac{c_{1}}{c_{2}}$ the given pair of equation are parallel to each other.

Comments

Tasneem

All Exercises - Chapter 3 - Pair of Linear Equations in Two Variable

Exercise 3.1

Exercise 3.2

Exercise 3.3

Exercise 3.4

Exercise 3.5

Exercise 3.6

Exercise 3.7

Feedback:

How helpful was it?

This article changed my life! This article was informative. I have a question.

How can we Improve it?

This article contains incorrect information. This article doesn't have the information I am looking for. I have a question.

Please tell us how it changed your life ^*

Please enter your feedback

Please enter your question below and we will send it to our tutor communities to answer it ^*

Please enter your question

Please select your tags

Please select a tag

Name ^*

Enter a valid name.

Email ^*

Enter a valid email.

Email or Mobile Number: ^*

Please enter your email or mobile number

Sorry, this phone number is not verified, Please login with your email Id.

Password: ^*

Please enter your password

By Signing Up, you agree to our Terms of Use & Privacy Policy

Thanks for your feedback

About UrbanPro

UrbanPro.com helps you to connect with the best Class 10 Tuition in India. Post Your Requirement today and get connected.

I am a Student I am a Tutor
Name*	Please enter your full name. Please enter institute name.
Email*	Please enter your email address.
Phone*	Please enter a valid phone number.
Location*	Please enter a pincode or area name.
City*	Please enter city name.
Category*	Please enter category.
Gender*	Male Female Please select your gender.
Email ID/ Mobile No.*	Please enter either mobile no. or email.
Enter Password*	Please enter OTP Please enter Password Sorry, this phone number is not verified, Please login with your email Id.

Learn Exercise 3.2 with Free Lessons & Tips

All Exercises - Chapter 3 - Pair of Linear Equations in Two Variable

About UrbanPro

Looking for Class 10 Tuition Classes?

Looking for Class 10 Tuition Classes?